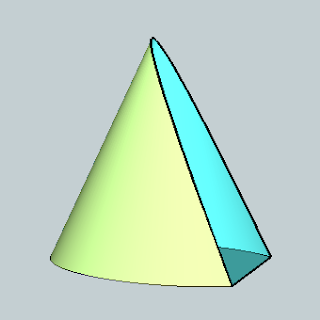
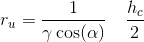Parabola by Conical Section
A parabola is created when a diagonal cutting plane passes through a right cone at an angle parallel to a side of the cone.
(In the images, imagine the cone extends downward to infinity, so the parabolic section created also goes to infinity.)
The height-to-base radius ratio of the cone is given by γ:
The angle of a side of the cone is, therefore, α = arctan(γ) with respect to the horizontal. To make a parabolic section, a cutting plane must pass through the cone at the same angle, α, with respect to the horizontal. Say this cutting plane is made such that it intersects the axis of the cone at a vertical distance hc from the tip of the cone.
rc is the radius of the cone where the cutting plane intersects the axis of the cone (= hc/γ).
ru is the distance from the cone axis to the upper edge of the cone, measured along the cutting plane.
In addition, an x-y-z coordinate system is affixed to the cutting plane.
(0, 0, 0) is on the cutting plane where it intersects the surface of the cone on the upper side.
Positive x is on the cutting plane, going through the cone axis and aligned with the long side of the cut.
Δ x = x - ru
Positive y is on the cutting plane, going up.
Positive z is perpendicular to the cutting plane.
Looking at the section from above, and perpendicular to, the plane of the cut, it appears as illustrated in the following image (note positive z comes out of the page toward at the viewer):
On the plane of the parabolic section, the distance from the cone axis to the upper edge of the cone is given by the same expression as for an elliptical section:
In fact, because the cutting plane is parallel to an edge of the cone when creating a parabola, the expression can be simplified somewhat:
or
or
The orientation of this example's geometry means the resulting parabola should take the following form:
One point on the parabola is already known, which can be used to solve for c:
ru = (1/(4c)) (rc)2
Solving for c:
or via the other two expressions for ru.
(Doing a quick comparison of ru and c, we see that ru is larger than c for most values of γ.)
At this point, we think and hope a parabolic section has been created. After all, that is what theory says, doesn't it? But let's confirm that theory for ourselves, and continue to work through an analysis of the physical model. We can confirm that a parabolic section is, indeed, produced by calculating coordinates along the cone/cutting plane intersection.
Each value of Δ x corresponds to a vertical displacement down the cone axis of (Δ x) (sin(α)) and at this point
h = hc + (Δ x) (sin(α))
The radius of the cone at this point is
r = [hc + (Δ x) (sin(α))] / γ
Looking at a circular cross-section of the cone at this point, the displacement perpendicular to the cone axis is (Δ x) (cos(α)). In the circular cross-section, this corresponds to an angle of
τ = arccos{[(Δ x) (cos(α))] / [r] }
The y-coordinate along the edge of the cone is then found as y = r sin(τ).
or
Now let's create a numeric example and generate some data.
Say γ = 1.27201964951 and hc = 100.
α = arctan(γ) = 0.904556894301 rad
ru = 63.6009824759
c = 24.2934135879
Tabulating values for x = 0 to x = 100, we get the following data.
These data points represent a parabolic curve with the c value stated above. Furthermore, these points are verified by the standard form for a parabola for the given value of c: x = (1/(4c)) y2
In other words, analysis of the physical model underlying a parabolic curve confirms the theory.
(In the images, imagine the cone extends downward to infinity, so the parabolic section created also goes to infinity.)
The height-to-base radius ratio of the cone is given by γ:
The angle of a side of the cone is, therefore, α = arctan(γ) with respect to the horizontal. To make a parabolic section, a cutting plane must pass through the cone at the same angle, α, with respect to the horizontal. Say this cutting plane is made such that it intersects the axis of the cone at a vertical distance hc from the tip of the cone.
rc is the radius of the cone where the cutting plane intersects the axis of the cone (= hc/γ).
ru is the distance from the cone axis to the upper edge of the cone, measured along the cutting plane.
In addition, an x-y-z coordinate system is affixed to the cutting plane.
(0, 0, 0) is on the cutting plane where it intersects the surface of the cone on the upper side.
Positive x is on the cutting plane, going through the cone axis and aligned with the long side of the cut.
Δ x = x - ru
Positive y is on the cutting plane, going up.
Positive z is perpendicular to the cutting plane.
Looking at the section from above, and perpendicular to, the plane of the cut, it appears as illustrated in the following image (note positive z comes out of the page toward at the viewer):
On the plane of the parabolic section, the distance from the cone axis to the upper edge of the cone is given by the same expression as for an elliptical section:
In fact, because the cutting plane is parallel to an edge of the cone when creating a parabola, the expression can be simplified somewhat:
or
or
The orientation of this example's geometry means the resulting parabola should take the following form:
One point on the parabola is already known, which can be used to solve for c:
ru = (1/(4c)) (rc)2
Solving for c:
or via the other two expressions for ru.
(Doing a quick comparison of ru and c, we see that ru is larger than c for most values of γ.)
At this point, we think and hope a parabolic section has been created. After all, that is what theory says, doesn't it? But let's confirm that theory for ourselves, and continue to work through an analysis of the physical model. We can confirm that a parabolic section is, indeed, produced by calculating coordinates along the cone/cutting plane intersection.
Each value of Δ x corresponds to a vertical displacement down the cone axis of (Δ x) (sin(α)) and at this point
h = hc + (Δ x) (sin(α))
The radius of the cone at this point is
r = [hc + (Δ x) (sin(α))] / γ
Looking at a circular cross-section of the cone at this point, the displacement perpendicular to the cone axis is (Δ x) (cos(α)). In the circular cross-section, this corresponds to an angle of
τ = arccos{[(Δ x) (cos(α))] / [r] }
The y-coordinate along the edge of the cone is then found as y = r sin(τ).
or
Now let's create a numeric example and generate some data.
Say γ = 1.27201964951 and hc = 100.
α = arctan(γ) = 0.904556894301 rad
ru = 63.6009824759
c = 24.2934135879
Tabulating values for x = 0 to x = 100, we get the following data.
| x | y | |
| 0 | 0 | |
| 10 | 31.1726890644 | |
| 20 | 44.0848396506 | |
| 30 | 53.9926812684 | |
| 40 | 62.3453781291 | |
| 50 | 69.7042517899 | |
| 60 | 76.3571821187 | |
| 63.6009824 | 78.615137776 | |
| 70 | 82.4751829621 | |
| 80 | 88.1696793012 | |
| 90 | 93.5180671937 | |
| 100 | 98.5766982364 |
These data points represent a parabolic curve with the c value stated above. Furthermore, these points are verified by the standard form for a parabola for the given value of c: x = (1/(4c)) y2
In other words, analysis of the physical model underlying a parabolic curve confirms the theory.
Labels: conical section, parabola, parabolic section